Exploding the Wente Torus

Constant mean curvature torus and cylinders in euclidean 3-space

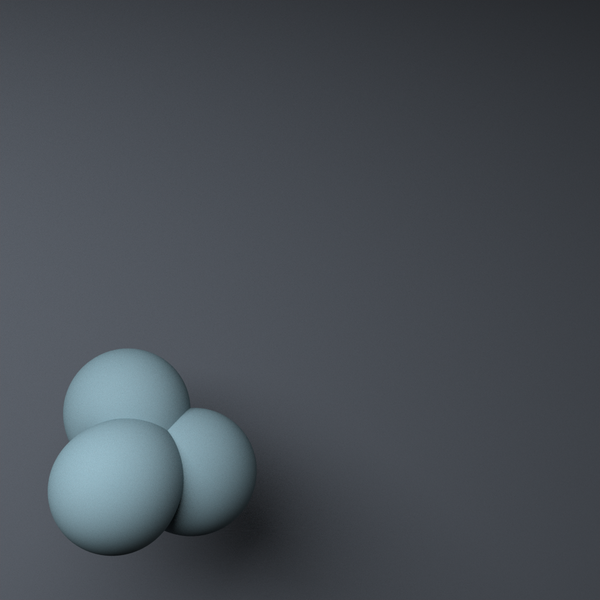
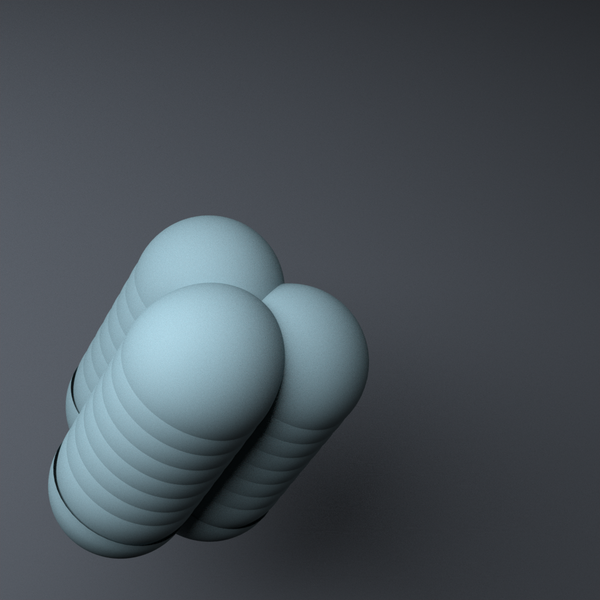
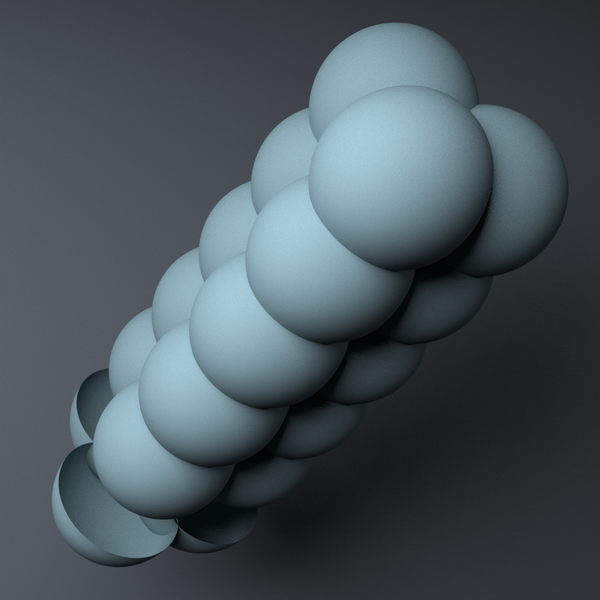
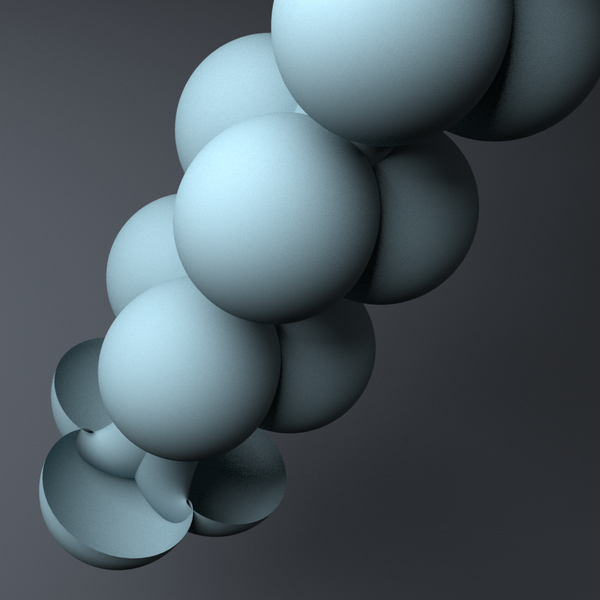
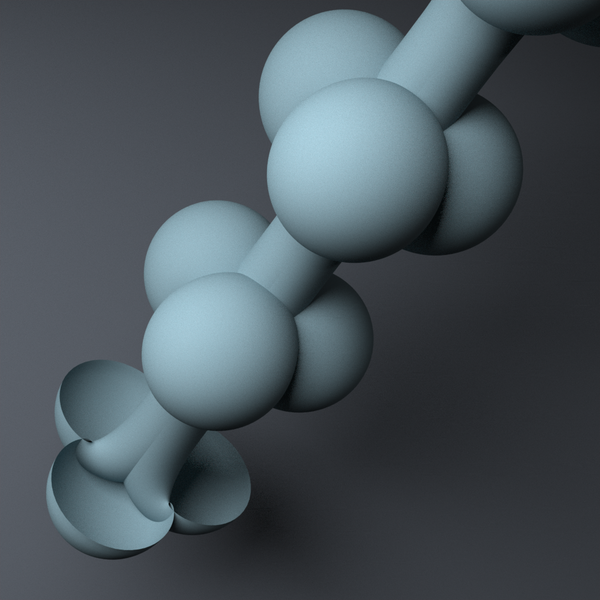
This sequence of images shows what happens when one of the periods of a Wente torus is broken: the surface explodes into a periodic bubbleton. The sequence was constructed by following a flow [3] through spectral genus 2 CMC cylinders, starting at a round cylinder. Two double points on the spectal curve were opened up to become branch points of the genus 2 spectal curve. [2,3,1].

The three-lobed Wente torus before being exploded.

One period is broken; the surface instantly becomes a cylinder.

The surface, which extends infinitely in both directions, is shown here cut off at both ends.

A 3-lobed periodic bubbleton. The continued flow increases the distance between the bubbles to infinity.

References

J. Bolton, F. Pedit, and L. Woodward, Minimal surfaces and the affine Toda field model, J. Reine Angew. Math. 459 (1995), 119—150 [1319519].
N. Hitchin, Harmonic maps from a 2-torus to the 3-sphere, J. Differential Geom. 31 (1990), no. 3, 627—710 [1053342].
M. Kilian and M. Schmidt, On the moduli of constant mean curvature cylinders of finite type in the 3-sphere, arXiv:0712:0108v2 (2008) link.
M. Kilian and M. Schmidt, On the moduli of constant mean curvature cylinders of finite type in the 3-sphere, arXiv:0712:0108v2 (2008) link.