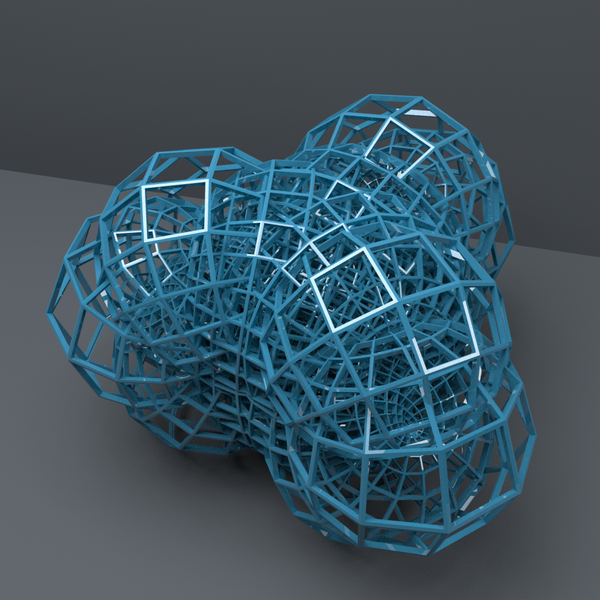

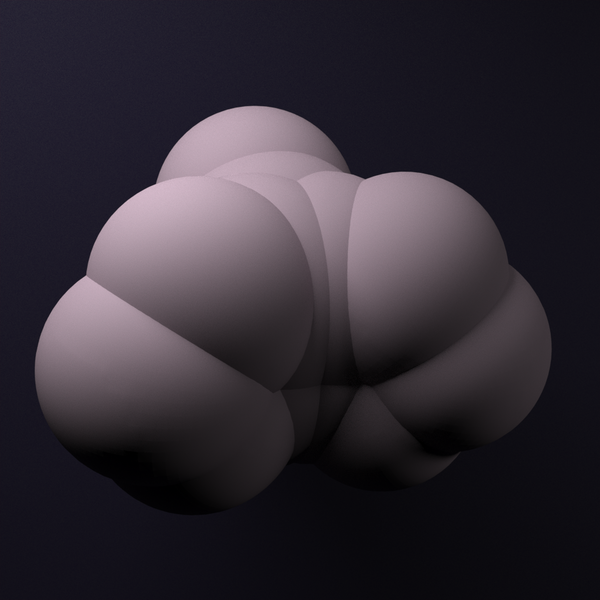
A constant mean torus in euclidean 3-space with spectral genus 3. The surface was constructed by following a period-preserving flow [3] through spectral genus 3 CMC tori in the 3-sphere, starting at a flat torus [2,3,1]. Three double points on the spectal curve were opened up to become branch points of the genus 3 spectal curve. The flow was continued until the mean curvature became infinite, limiting to a CMC surface in euclidean 3-space.


